Nonsmooth Dynamical Systems with Noise
I am currently studying for my PhD in the School of Mathematics, Statistics and Applied Mathematics at NUI Galway, studying nonsmooth dynamical systems with stochastic noise under the supervision of Dr Petri Piiroinen funded by a Government of Ireland Postgraduate Scholarship from the Irish Research Council.
Historically mathematicians and scientists have made widespread use of smooth,
deterministic mathematical models to describe real-world phenomena. These models
present a simplified view of the world where, on one hand, the evolution of systems is always
smooth and exhibits no interruptions such as impacts, switches, slides or jumps and, on the
other hand, the future of any system is completely determined by its present state.
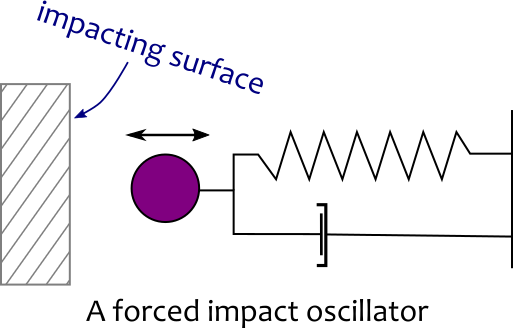
However, when modelling many real-world systems one or both of these simplifications may not hold. For example, mechanical systems involving impacts or friction and electrical systems with switches behave in a nonsmooth manner and more complex systems such as the world's climate and financial systems have also been modelled using nonsmooth models. Furthermore, real-world systems are also rarely entirely deterministic and it has been shown that a level of randomness or noise is ubiquitous in real-world systems. Models known as stochastic models possess some inherent randomness, allowing us to account for this noise.
Independently, both noise and nonsmoothness have been shown to be the drivers of
significant changes in qualitative behaviour. In nonsmooth systems we find certain types of
qualitative changes in the behavior of the system under parameter variation that do not occur
in the smooth setting. Adding noise to smooth systems has been shown to have the potential
to do far more than just blur the outcome of the system in the absence of noise, in some
cases even inducing stability in unstable systems or destabilising stable systems.
This project investigates systems which are both nonsmooth and noisy, which have so far
seen very limited research. In particular we wish to establish how the features of nonsmooth
systems can be affected by the addition of noise and how these effects relate to real-world
systems in areas such as climate change, finance and mechanics.
Dynamic Spatial Networks and Economic Development
I also completed my MSc in the School of Mathematics, Statistics and Applied Mathematics at NUI Galway under the supervision of Dr Petri Piiroinen from the School of Mathematics, Statistics and Applied Mathematics, Dr Srinivas Raghavendra from the College of Business, Public Policy and Law and Dr Jim Duggan from the College of Engineering and Informatics. This applied mathematics project with interdisciplinary elements introduced a set of spatial network growth models which allow for the growth of both the network and the individual nodes within the network and applied the ideas developed to problems related to spatial economic development.
The spatial agglomeration of economic activities and the unequal growth of urban centres are major topics of interest to both economists and policymakers worldwide. In this project we aim to model and analyse these uneven spatial economic dynamics by setting up an agent-based simulation environment. Our approach is to build mathematical models composed of multiple overlapping dynamic spatial networks across space and time. We introduce our approach to modelling the evolution and growth of spatial networks, both in terms of individual node sizes and the addition of links and nodes to the network. Motivated by official statistics related to the economy of Ireland we then apply the ideas developed in an attempt to model spatial economic dynamics in a small open economy.
I am currently in the process of preparing two articles for publication based on this work.
The Jacobi Equation and Manifolds with Multiple Conjugate Points
During a Summer intership in the School of Mathematics, Statistics and Applied Mathematics at NUI Galway in 2012 I carried out a research into the phenomenon of multiple conjugate points along a geodesic under my supervisor Dr John Burns.
We investigated conjugate points in the context of the Jacobi equation, a second order ordinary differential equation, which captures precisely the geometry of conjugate points on surfaces. The results were published in the Mathematical Proceedings of the Royal Irish Academy. Link